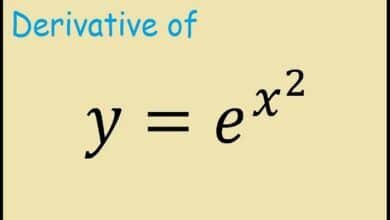
Introduction
The domain of a function, denoted as f(x), is a fundamental concept in mathematics, especially in algebra and calculus. Understanding the domain of f(x) is crucial for solving equations, analyzing graphs, and applying functions in real-world scenarios. This comprehensive guide will walk you through the intricacies of the domain of f(x), ensuring you grasp its importance and applications.
What is the Domain of f(x)?
The domain of f(x) refers to all possible input values (x-values) for which the function f(x) is defined. It essentially answers the question: “For what values of x does this function produce a valid output?”
Why is the Domain of f(x) Important?
Domain of f(x) is important because it helps in determining the range of values over which the function operates. This knowledge is crucial for graphing functions, solving equations, and avoiding undefined expressions such as division by zero.
Basic Concepts: Definitions and Examples
In simple terms, the domain of f(x) includes all real numbers unless restrictions are specified. For example, the domain of f(x) = 2x + 3 is all real numbers because there are no restrictions on x.
Determining the Domain of f(x) in Rational Functions
Rational functions have the form f(x) = P(x)/Q(x), where P(x) and Q(x) are polynomials. The domain of f(x) in rational functions excludes values that make the denominator Q(x) equal to zero, as division by zero is undefined.
The Domain of f(x) in Radical Functions
For radical functions, such as f(x) = √(x-2), the domain of f(x) includes values of x that make the expression inside the radical non-negative. For instance, in f(x) = √(x-2), x must be greater than or equal to 2.
The Domain of f(x) in Logarithmic Functions
Logarithmic functions, like f(x) = log(x-1), have a domain of f(x) where the argument of the logarithm (x-1) must be positive. Therefore, x must be greater than 1 for the function to be defined.
Piecewise Functions and Their Domains
Piecewise functions are defined by different expressions for different parts of their domain. For each piece of the function, you need to consider the specific conditions that define the domain of f(x) for that segment.
Finding the Domain of Composite Functions
Composite functions involve applying one function to the results of another, denoted as (f∘g)(x). The domain of f(x) in composite functions requires ensuring that the domain of g(x) fits within the domain of f(x).
Graphical Representation of the Domain of f(x)
Graphing a function can help visualize its domain. The domain of f(x) is represented on the x-axis, and identifying points where the function is not defined can clarify the domain restrictions.
Real-World Applications of the Domain of f(x)
In real-world scenarios, understanding the domain of f(x) is vital in fields like engineering, physics, and economics. For instance, in physics, the domain of f(x) might represent time intervals during which a physical phenomenon occurs.
Conclusion
The domain of f(x) is a critical concept that underpins much of mathematical analysis and application. By understanding and determining the domain of f(x), you can effectively solve equations, graph functions, and apply mathematical principles to real-world problems. Whether dealing with polynomials, rational functions, radicals, or logarithms, knowing the domain of f(x) is essential for ensuring valid and meaningful results.
FAQs
1. How do you determine the domain of f(x) for a rational function?
To determine the domain of f(x) for a rational function, identify the values that make the denominator zero and exclude them from the domain.
2. What is the domain of f(x) = √x?
The domain of f(x) = √x is x ≥ 0 because the square root function is defined only for non-negative values.
3. How does the domain of f(x) change for a piecewise function?
For a piecewise function, the domain of f(x) is determined by the combined intervals of all the pieces, ensuring each segment’s conditions are met.
4. Can the domain of f(x) include negative numbers?
Yes, the domain of f(x) can include negative numbers unless restricted by the function’s form, such as in the case of square roots or logarithms where the input must be positive.
5. What is the significance of the domain of f(x) in calculus?
In calculus, the domain of f(x) is significant because it defines the range of input values over which you can differentiate and integrate the function, impacting the analysis and solutions of calculus problems.