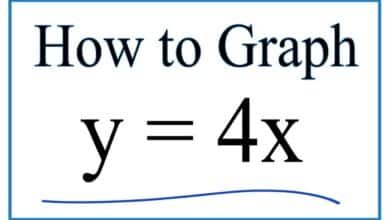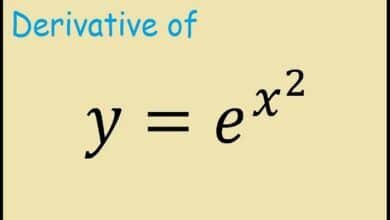
When you encounter f(x)f(x)f(x) in mathematics, you’re looking at a function. This symbol is a fundamental concept across various branches of math and science, representing a special relationship between sets of numbers. In this post, we will explore what f(x)f(x)f(x) means, its significance, and its applications in real-world scenarios.
What Does f(x)f(x)f(x) Represent?
f(x)f(x)f(x) denotes a function named ‘f’ with ‘x’ as its independent variable. This notation helps in expressing how ‘x’ is mapped to another value, often referred to as ‘y’ or f(x)f(x)f(x). This mapping indicates that for every ‘x’, there is a corresponding f(x)f(x)f(x).
Types of Functions
There are various types of functions in mathematics, including linear, quadratic, polynomial, and trigonometric functions. Each type has a unique way of relating xxx to f(x)f(x)f(x), which dictates the graph’s shape and the function’s properties.
Function Notation and Its Importance
Function notation, such as f(x)f(x)f(x), is crucial because it provides a clear and concise way to describe the relationship between variables. This notation is not just symbolic but is a powerful tool in calculus, algebra, and beyond.
How to Evaluate f(x)f(x)f(x)
Evaluating f(x)f(x)f(x) involves substituting a number for xxx and calculating the result. This process is essential for solving equations, predicting outcomes, and understanding behavior within a given domain.
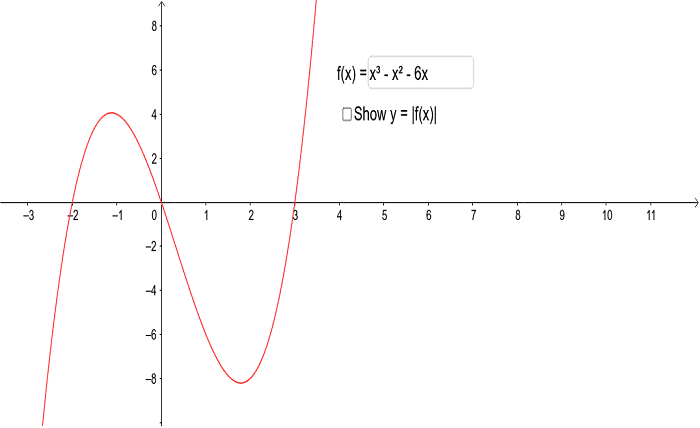
Graphing f(x)f(x)f(x)
Graphing f(x)f(x)f(x) helps visualize the relationship between xxx and f(x)f(x)f(x). It’s a vital step in understanding the function’s behavior, identifying its maxima, minima, and trends.
f(x)f(x)f(x) in Calculus
In calculus, f(x)f(x)f(x) plays a central role. It is used to define limits, derivatives, and integrals, which are foundational concepts for understanding change and area under curves.
Applications of f(x)f(x)f(x)
f(x)f(x)f(x) is not limited to theoretical math; it has practical applications in physics, engineering, economics, and data science. Understanding functions allows professionals to model and solve real-world problems effectively.
f(x)f(x)f(x) in Programming
In programming, functions are blocks of code designed to perform specific tasks, much like mathematical functions. They take inputs, process them, and return outputs. Understanding f(x)f(x)f(x) can enhance logical thinking and problem-solving skills in programming.
Common Misconceptions About f(x)f(x)f(x)
Many students confuse f(x)f(x)f(x) with being a product of fff and xxx. However, it’s crucial to understand that f(x)f(x)f(x) represents the output value of the function fff at a particular xxx.
Conclusion
f(x)f(x)f(x) is crucial for students and professionals alike, as it forms the basis of many advanced topics in mathematics and science. By mastering how to work with functions, you can unlock new opportunities in analytical thinking and problem-solving.
FAQs
- What does f(x)=x2f(x) = x^2f(x)=x2 represent?
- This represents a quadratic function where the output is the square of the input. It’s graphed as a parabola.
- Can f(x)f(x)f(x) have more than one value for a single xxx?
- No, in standard function notation, each input xxx has exactly one output f(x)f(x)f(x).
- Is f(x)f(x)f(x) always a formula?
- While often expressed as a formula, f(x)f(x)f(x) can also be represented using a graph, a table, or verbally described relationships.
- How does f(x)f(x)f(x) differ from g(x)g(x)g(x)?
- f(x)f(x)f(x) and g(x)g(x)g(x) are simply different functions or different rules for relating xxx to an output. They can have different formulas, properties, and graphs.
- Can f(x)f(x)f(x) be used in everyday life?
- Absolutely. Functions model relationships between variables, useful in fields like finance for calculating interest, in science for describing physical laws, or in technology for programming.