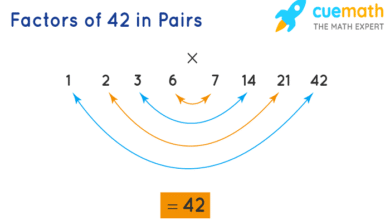
The number 80 is more than just a figure; it’s a cornerstone in various mathematical and real-world applications. From its role in timekeeping (seconds in a minute and a third) to its presence in geometry and algebra, understanding the factor pairs of 80 can enhance our grasp of mathematics and its practical implications. This blog post delves into the factor pairs of 80, exploring each in detail and unveiling their unique properties and significance.
Factor Pairs
Before diving into the specific factor pairs of 80, it’s crucial to grasp what factor pairs are. A factor pair of a number consists of two integers that, when multiplied together, produce the original number. This section explains the concept of factors and how to determine them for any given number.
The Significance of Factor Pairs in Mathematics
Factor pairs are fundamental in various branches of mathematics, including algebra, number theory, and geometry. This part discusses how factor pairs are used to simplify equations, solve problems, and understand numerical relationships.
Factor Pair of 80: 1 and 80
Every number has at least one factor pair that includes the number itself and one. This segment explores how the factor pair (1, 80) relates to the properties of 80, including its role as a highly composite number.
Factor Pair of 80: 2 and 40
The factor pair (2, 40) demonstrates the relationship between even numbers and their divisibility by 2. This section examines the implications of this factor pair in real-world contexts and mathematical theory.
Factor Pair of 80: 4 and 20
Delving into the factor pair (4, 20), this part discusses its occurrence in everyday life and its mathematical significance, particularly in measurements and fractions.
Factor Pair of 80: 5 and 16
The factor pair (5, 16) is intriguing due to its involvement with pentagonal and hexadecimal systems. This section explores the geometric and numeric significance of this pair.
Factor Pair of 80: 8 and 10
Highlighting the factor pair (8, 10), this part focuses on its applications in digital technology and common metric measurements, reflecting on how these factors are used in computing and architecture.
Practical Applications of 80’s Factor Pairs
Factor pairs of 80 have practical applications in real life, including technology, engineering, and education. This section illustrates how these factor pairs simplify computations and enhance efficiency in various fields.
Factor Pairs and Prime Factorization
Understanding the prime factors of 80 helps in comprehending its factor pairs. This part breaks down the prime factorization of 80 and links it to the concept of factor pairs, explaining how this knowledge is useful in advanced mathematics.
Educational Importance of Learning Factor Pairs
Why is it important for students to learn about factor pairs? This section discusses the educational benefits of understanding factor pairs, including problem-solving skills and foundational mathematical concepts.
Challenges and Puzzles Involving Factor Pairs of 80
Engage with some mathematical puzzles and challenges that involve the factor pairs of 80. This fun section tests your understanding and application of the concepts discussed throughout the post.
Conclusion
The factor pairs of 80 offer a fascinating glimpse into the world of mathematics, providing a foundational understanding that applies to both theoretical concepts and practical applications. By exploring these pairs, we gain insights into the structure and properties of numbers that influence various aspects of daily life and scientific inquiry.
FAQs
1.What are factor pairs?
Factor pairs are two numbers that, when multiplied together, produce a given product. For the number 80, its factor pairs are pairs of numbers that multiply to give 80.
2.How many factor pairs does 80 have?
The number 80 has five factor pairs: (1, 80), (2, 40), (4, 20), (5, 16), and (8, 10).
3.Why are factor pairs important in mathematics?
Factor pairs are crucial in simplifying algebraic expressions, solving equations, and understanding number properties, which are essential in higher mathematical learning and applications.
4.Can understanding factor pairs help in real-life situations?
Yes, understanding factor pairs can help in various real-life applications such as computing, architecture, and time management, where quick calculations and divisions are needed.
5.Are there any educational tools to help learn about factor pairs? \
Educational tools such as multiplication tables, online calculators, and interactive math games can significantly aid in learning about factor pairs and their applications.